Bifurcations

Next, we need to understand how fixed points and periodic orbits change as parameters are varied. It is possible that at some particular set of parameters a fixed point or periodic orbit is stable, but at a different set it is unstable. Such a change in stability under a change in parameters is an example of a bifurcation. Another example of a bifurcation is when, as parameters are changed, new fixed points or periodic orbits come into existence. Loosely speaking, a bifurcation is a qualitative change in the dynamics of the system of ODEs as a parameter varies. We now give several examples of bifurcations. We show the flow of the vector fields in phase space, that is, the space of the variables ${\bf x}$. The bifurcation diagrams summarize the behavior near the bifurcation; solid lines show stable solutions, while dashed lines show unstable solutions.

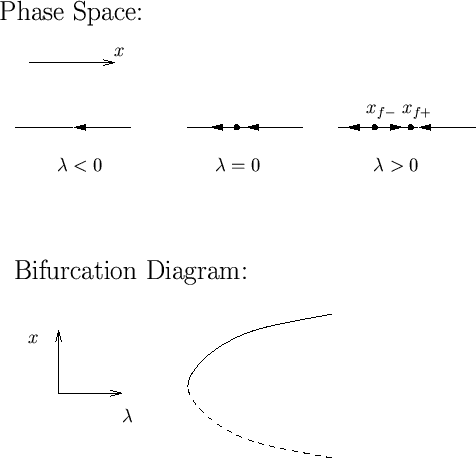
Saddlenode bifurcation: Consider the one-dimensional differential equation

$$\dot{x} = \lambda - x^2.$$ (2)

It is readily shown that no fixed points exist for $\lambda<0$, but fixed points $x_{f\pm} = \pm \sqrt{\lambda}$ exist for $\lambda \ge 0$. $x_{f+}$ is stable, while $x_{f-}$ is unstable. The qualitative change in behavior at $\lambda=0$ is called a saddlenode bifurcation.

Figure 1: Top: Fixed points and flow for equation (2). Bottom: Bifurcation diagram for the saddlenode bifurcation.

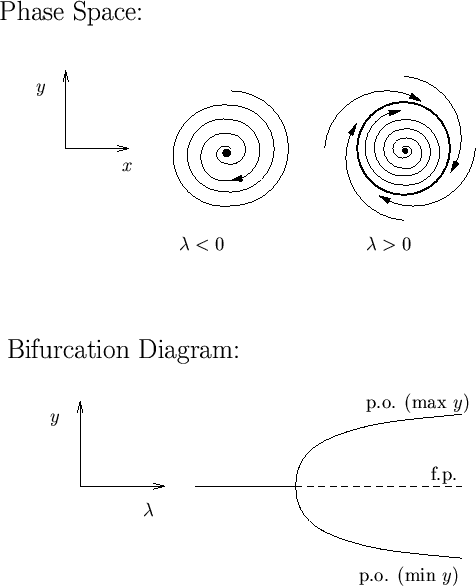
Hopf bifurcation: A Hopf bifurcation involves the change in stability of a fixed point of a dynamical system together with the birth of a periodic orbit. For example, for the Hopf bifurcation shown in Figure 2, for $\lambda<0$ there is a stable fixed point, while for $\lambda>0$ there is an unstable fixed point and a stable periodic orbit.

Figure 2: Top: Phase space for an example of a Hopf bifurcation. Bottom: Bifurcation diagram for the Hopf bifurcation. In this diagram, both the maximum and the minimum values of $y$ on the periodic orbit are shown.

Homoclinic bifurcation: A homoclinic orbit is a trajectory which approaches a fixed point both as $t \rightarrow \infty$ and as $t \rightarrow -\infty$. The formation of a homoclinic orbit as a parameter is varied, called a homoclinic bifurcation, can lead to the creation or destruction of a periodic orbit. For the homoclinic bifurcation shown in Figure 3, a homoclinic orbit forms at $\lambda=0$, and a periodic orbit exists for $\lambda>0$ but not for $\lambda<0$. As $\lambda \rightarrow 0^+$, the period $T$ of the periodic orbit diverges to infinity.

Figure 3: Top: Phase space and bifurcation diagram for a homoclinic bifurcation giving birth to a stable periodic orbit as $\lambda$ increases through 0. The open dots show fixed points which do not participate in the bifurcation. Bottom: Bifurcation diagram showing the period of the periodic orbit as a function of $\lambda$.