


Next: An SIS model
Up: APC/EEB/MOL 514 Tutorial 4:
Previous: APC/EEB/MOL 514 Tutorial 4:
In this computer lab we'll consider some generalizations to the
epidemiological models covered in the lectures. The motivation for these
generalizations will be the fact that for some diseases the number of
infected individuals 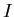 in a population oscillates seasonally. For
example, childhood diseases such as measles and rubella increase each
winter because of increased contact between children at school.
Specifically, we'll explore what happens when the contact rate is a
periodic function of time. It will be shown for a simple SIS model
(which is very similar to the SIR model covered in lecture except
that recovered individuals return to class S instead of passing to class
R) that such a periodic contact rate can lead to a periodic oscillation
in
in a population oscillates seasonally. For
example, childhood diseases such as measles and rubella increase each
winter because of increased contact between children at school.
Specifically, we'll explore what happens when the contact rate is a
periodic function of time. It will be shown for a simple SIS model
(which is very similar to the SIR model covered in lecture except
that recovered individuals return to class S instead of passing to class
R) that such a periodic contact rate can lead to a periodic oscillation
in 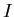 with the same period as the contact rate. For more complicated
models, it is possible to get states in which the period of
with the same period as the contact rate. For more complicated
models, it is possible to get states in which the period of 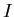 is some
integer multiple of the period of the contact rate. In fact, as
parameters are varied it is possible for some models to get a period
doubling cascade to chaotic behavior.
is some
integer multiple of the period of the contact rate. In fact, as
parameters are varied it is possible for some models to get a period
doubling cascade to chaotic behavior.



Next: An SIS model
Up: APC/EEB/MOL 514 Tutorial 4:
Previous: APC/EEB/MOL 514 Tutorial 4:
Jeffrey M. Moehlis
2002-10-14